[Book Note] Ch 3. Some Properties of the Euler Equations (1)

One-Dimensional Euler Equations

• Euler equation은 압축성 medium에 대한 body force, viscosity, heat conduction 영향을 무시함으로써 얻을 수 있음.
• Conservative와 non-conservative 수식을 활용하여 이상기체 EOS에 대한 1차원 time-dependent Euler equations를 얻을 수 있음.

 

Conservative Formulation

• Euler equation의 conservative formulation을 differential form으로 표현하면 다음과 같음. 
  $$ \mathbf{U}_t + \mathbf{F(U)}_x = 0, $$$$ \mathbf{U} = [\rho, \rho u, E]^\text{T}, \quad \mathbf{F} = [\rho u, \rho u^2 + p, u(E+p)]^\text{T} $$
• 이를 quasi-linear form으로 표현하면 다음과 같음. $$ \mathbf{U}_t + \mathbf{A(U)U}_x = 0, \quad \mathbf{A(U)}=\partial\mathbf{F} / \partial\mathbf{U} $$

Proposition: Homogeneity Property

• Ideal-gas EOS가 적용된 Euler equation는 *homogeneity property $\mathbf{F(U)}=\mathbf{A(U)U}$를 만족함.

*Flux Vector Splitting의 numerical scheme 기반이 됨.

Proposition: Hyperbolic Equation

• Euler equation의 jacobian matrix에 대해서 eigenvalues와 right eigenvector는 다음과 같음. 
  $$ \lambda_1 = u-a, \quad \lambda_2 = u, \quad \lambda_3 = u+a $$$$ \mathbf{K}^\text{(1)} = [1, u-a, H-ua]^\text{T}, \quad \mathbf{K}^\text{(2)} = [1, u, 0.5u^2]^\text{T}$$ $$ \mathbf{K}^{(3)} = [1, u+a, H+ua]^\text{T} $$
  • Eigenvalues가 all real이고, linearly independent eigenvectors의 complete set을 형성하기 때문에 이상기체에 대한 time-dependent, one-dimensional Euler equation는 hyperbolic, strictly hyperbolic ($\because$ sound speed $a$가 양수이면 eigenvalue는 real이자 distinct).

 

Non-Conservative Formulations

• Euler equation은 conservative variables이 아닌 other variables로 표현할 수 있으며, smooth solution에 대해서는 모두 동일함. 하지만, shock wave가 포함되어 있는 경우, non-conservative로 표현된 Euler equation은 부정확한 shock solution을 도출함.
• Equation을 분석할 때는 non-conservative 식이 conservative 식보다 이점이 있음.

Primitive-Variable Formulation

• Smooth solution일 때, conservative variable 대신 primitive (or physical) variables $\mathbf{W} =[\rho, u, p]^\text{T}$로 formulation 할 수 있음. Quasi-linear form으로 표현하면 다음과 같음.

$$ \mathbf{W}_t + \mathbf{A(W)W}_x = 0 $$

$$ \mathbf{W} = [\rho, u, p]^\text{T} $$

\[
\mathbf{A(W)} =
\begin{bmatrix}
u & \rho & 0 \\
0 & u & 1/\rho \\
0 & \rho a^2 & u
\end{bmatrix}
\]

Characteristic Equations

• Primitive variable로 표현된 Euler equation의 eigenvalues와 eigenvectors는 다음과 같음.
  $$ \lambda_1 = u-a, \quad \lambda_2 = u, \quad \lambda_3 = u+a $$ $$ \mathbf{K}^{(1)} = \alpha_1[1, -a/\rho, a^2]^\text{T}, \quad \mathbf{K}^{(2)} = \alpha_2[1, 0, 0]^\text{T}, $$ $$ \mathbf{K}^{(3)} = \alpha_3[1, a/\rho, a^2]^\text{T} $$ $$ \mathbf{L}^{(1)} = \beta_1(0, 1, -1/\rho a) $$ $$ \mathbf{L}^{(2)} = \beta_2(1, 0, -1/a^2) $$ $$ \mathbf{L}^{(3)} = \beta_3(0, 1, 1/\rho a) $$
  • Characteristic equation은 $dx/dt = \lambda_i$ 방향으로 $\mathbf{L}^{(i)} \cdot d\mathbf{W} = 0$를 만족하기 때문에, 아래와 같이 전개하여 characteristic equation을 얻을 수 있음.
    $$ \mathbf{L}^{(i)} \cdot [d\rho, du, dp]^\text{T} = 0$$ \[
    \begin{cases}
    dp - \rho a du = 0 \quad \text{along} \quad dx/dt = \lambda_1 = u - a, \\
    dp - a^2 d \rho = 0 \quad \text{along} \quad dx/dt = \lambda_2 = u, \\
    dp + \rho a du = 0 \quad \text{along} \quad dx/dt = \lambda_3 = u + a.
    \end{cases}
    \]
  • 이 *differential relation은 characteristic direction을 따라 유효함. 

*Numerical 목적으로, 이 식들의 선형화는 Euler equation에 대한
Riemann problem을 approximately 해결하는 방법을 제시함.

Entropy Formulation

• Pressure을 entropy $s$로 표현하여, 이전의 primitive-variable formulation을 $\mathbf{W}=(\rho, u, s)^\text{T}$로 표현할 수 있음.
  $$ s = c_v \ln\frac{p}{\rho^\gamma} + C_0 $$ \[ p = C_1 \rho^\gamma e^{s / c_v} \]
  • Entropy $s$는 $s_t+us_x=ds/dt=0$을 만족하는데, 이는 smooth flow region에서 entropy가 particle path $dx/dt=u$를 따라서 일정하다는 것을 의미함. 따라서 particle path를 따라, isentropic law $p=C\rho^\gamma$가 성립함.
    • $C=C(s_0)$는 initial entropy $s_0$의 함수이며, flow가 smooth하면 path를 따라 일정한 값을 가짐. 만약 $C$가 flow domain에 걸쳐서 일정하다면, 이를 isentropic flow 또는 homentropic flow라고 함.
• Entropy formulation은 다음과 같이 quasi-linear form으로 표현할 수 있음.
  $$ \mathbf{W}_t + \mathbf{A(W)W}_x = 0 $$ $$ \mathbf{W} = [\rho, u, s]^\text{T} $$ \[ \mathbf{A(W)} =
  \begin{bmatrix}
  u & \rho & 0 \\
  a^2/\rho & u & \frac{1}{\rho} \frac{\partial p}{\partial s} \\
  0 & 0 & u
  \end{bmatrix}
  \]
  • Eigenvalues와 eigenvector는 다음과 같음. $$ \lambda_1 = u-a, \quad \lambda_2 = u, \quad \lambda_3 = u+a $$ $$ \mathbf{K}^{(1)} = [1, -a/\rho, a^2]^\text{T}, \quad \mathbf{K}^{(2)} = [-\partial p/\partial s, 0, a^2]^\text{T}, $$ $$ \mathbf{K}^{(3)} = [1, a/\rho, 0]^\text{T} $$

 

Elementary Wave Solutions of the Riemann Problem

• Riemann problem를 물리적으로는 gas dynamics에서의 shock-tube problem으로 생각할 수 있음.

• Time-dependent, one dimensional Euler equation의 solution은 3개의 characteristic fields와 관련된 3개의 waves로 이루어져 있음. 
  • 3개의 wave는 4개의 constant state $(\mathbf{U_\text{L}} \rightarrow \mathbf{U_\text{*L}} \rightarrow \mathbf{U_\text{*R}} \rightarrow \mathbf{U_\text{R}})$로 분리함
    

    

  • Solution의 waves는 rarefaction waves, contact discontinuities, shock waves의 3가지 형태 중 하나임. 형태를 확인하기 위해 characteristic field $\mathbf{K}^{(i)}, \ i=1,2,3$를 분석해야 함.
    • $\mathbf{K}^{(2)}$-characteristic field는 linearly degenerate $\rightarrow$ contact discontinuity
      • Contact wave의 경우, characteristics가 contact wave에 평행함. $$ \lambda_2(\mathbf{U}_{*L}) = \lambda_2(\mathbf{U}_{*R}) = S_2 $$
    • $\mathbf{K}^{(1)}, \mathbf{K}^{(3)}$-characteristic field는 genuinely non-linear $\rightarrow$ rarefaction waves (smooth) or shock waves (discontinuities).
      
      • Rarefaction wave의 경우, eigenvalue $\lambda_1(\mathbf{U})$가 rarefaction wave를 왼쪽에서 오른쪽으로 지나면서 monotonically 증가함. 그리고 양단의 characteristics는 wave에서 diverge 함.
        $$ \lambda_1(\mathbf{U_\text{L}}) \le \lambda_1(\mathbf{U}_\text{*L}) $$
      • Shock wave의 경우, characteristics가 wave로 모이며 아래의 entropy condition을 만족함. \[
        \lambda_3(\mathbf{U}_{*R}) > S_3 > \lambda_3(\mathbf{U}_{R})
        \]

 

 

 

 

Contact Discontinuities

• Euler equation의 contact discontinuity는 equation의 eigenstructure를 활용하여 분석할 수 있음. 아래의 Generalised Riemann Invariants를 통해 contact wave를 건너며 pressure와 velocity가 일정하다는 것을 알 수 있음. $$ \frac{d\rho}{1} = \frac{d(\rho u)}{u} = \frac{dE}{0.5u^2} $$
  • Contact wave는 discontinuous wave이며, 이를 가로지르면서 pressure와 particle velocity는 일정하지만, density는 discontinuously jump 하고, density에 의존하는 specific internal energy, temperature, sound speed, entropy 등의 variables도 discontinuously jump함.  
• Primitive-variable formulation의 eigenvector $\mathbf{K}^{(2)}$를 통해서도 동일한 결과를 얻을 수 있음. $$ \mathbf{W} = [\rho, u, p]^\text{T}, \quad \mathbf{K}^{(2)} = [1, 0, 0]^\text{T} $$
  • $\rho, u, p$의 wave jump는 eigenvector의 corresponding component에 비례함. 속도와 압력에 대해서 0의 값을 가지고, $\rho$의 jump는 non-trivial임. 

Rarefaction Waves

• Euler equation에서의 rarefaction wave는 $\mathbf{K}^{(1)}, \mathbf{K}^{(3)} $ characteristic fields와 연관됨. primitive-variables formulation에 대한 eigenvector을 통해서, $\rho, u, p$가 rarefaction wave를 지나면서 변화함을 확인할 수 있음.

$$ \mathbf{K}^{(1)} = \alpha_1[1, -a/\rho, a^2]^\text{T}, \quad  \mathbf{K}^{(3)} = \alpha_3[1, a/\rho, a^2]^\text{T} $$

• Entropy formulation의 eigenstructure에 대한 Generalised Riemann Invariants를 통해서 아래 식을 유도할 수 있음.
  \[
  \begin{cases}
  I_\text{L}(u, a) = u + \frac{2a}{\gamma - 1} = \text{constant}\\
  s = \text{constant}
  \end{cases}
  \quad \text{across} \quad \lambda_1 = u - a,
  \]\[
  \begin{cases}
  I_\text{R}(u, a) = u - \frac{2a}{\gamma - 1} = \text{constant}\\
  s = \text{constant}
  \end{cases}
  \quad \text{across} \quad \lambda_3 = u + a,
  \]
  • Rarefaction wave는 1, 3 field와 관련된 smooth wave이며, 이를 가로지르면서 density, velocity, pressure가 변화함. Wave는 fan-type shape을 가지며, wave의 Head와 Tail에 상응하는 bouding characteristics에 의해 닫힘. 

Shock Waves

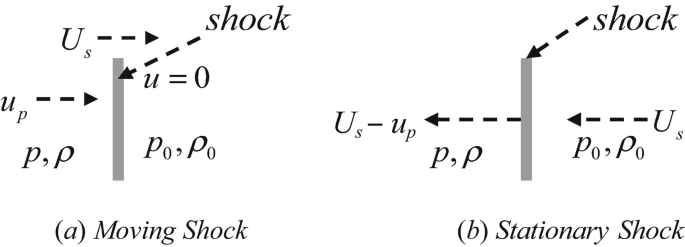
• Euler equation에서의 shock wave는 genuinely non-linear field 1, 3과 연관된 discontinuous wave이며, $\rho, u, p$가 shock wave를 건너면서 변화함. 
  • $\mathbf{K}^{(3)}$ characteristic field와 그에 상응하는 wave가 constant speed $S_3$의 right-facing shock wave이라고 하면, shock 이전의 state를 $\mathbf{W}_\text{R} = (\rho_R, u_R, p_R)^\text{T}$로, 이후 state를 $\mathbf{W}_{*} = (\rho_{*}, u_{*},  p_{*} )^\text{T}$로 표현할 수 있음.
  • 여기서, shock speed가 0이 되도록 문제를 변환하는 것이 유용하며, 이에 따라, stationary shock에서 shock의 앞, 뒤 state도 변화하게 됨. Density와 pressure는 바뀌지 않고 그대로 유지되지만, velocity는 아래와 같이 relative velocity $\hat{u}_\text{R}, \hat{u}_{*}$로 변화함.$$ \hat{u}_{*} = u_{*} - S_3, \quad \hat{u}_\text{R} = u_\text{R} - S_3 $$
  • Shock speed가 0인 frame에서의 Rankine-Hugoniot condition을 적용하면 다음과 같이 식을 정리할 수 있음.$$ \rho_*\hat{u}_* = \rho_\text{R}\hat{u}_\text{R}, $$ $$ \rho_*\hat{u}_*^2 + p_* = \rho_\text{R}\hat{u}^2_\text{R} + p_\text{R}, $$ $$ \hat{u}_*(\hat{E}_* + p_*) = \hat{u}_\text{R}(\hat{E}_\text{R} + p_\text{R}) $$
    • Total energy를 specific internal energy $e$로 표현하면 다음과 같음.$$ \hat{u}_* \rho_*\left[ \frac{1}{2}\hat{u}^2_* + (e_* + p_*/\rho_*) \right] = \hat{u}_\text{R} \rho_\text{R}\left[ \frac{1}{2}\hat{u}^2_\text{R} + (e_\text{R} + p_\text{R}/\rho_\text{R} ) \right] $$
    • Specific enthalpy $h$를 사용하고 식들을 조합하여 정리하면, 아래와 같이 표현할 수 있음. $$ \hat{u}^2_* = \left( \frac{\rho_\text{R}}{\rho_*} \right) \left[ \frac{p_\text{R} - p_*}{\rho_\text{R} - \rho_*} \right] $$ $$ \hat{u}^2_\text{R} = \left( \frac{\rho_*}{\rho_\text{R}} \right) \left[ \frac{p_\text{R} - p_*}{\rho_\text{R} - \rho_*} \right] $$ \[
      h_* - h_\text{R} = \frac{1}{2}(p_* - p_\text{R})\left[ \frac{\rho_* + \rho_\text{R}}{\rho_* \rho_\text{R}} \right]
      \]
    • Specific internal energy $e$가 caloric equation of state로 주어지면, 아래와 같이 표현할 수 있음. \[
      e_* - e_\text{R} = \frac{1}{2}(p_* + p_\text{R})\left[ \frac{\rho_* - \rho_\text{R}}{\rho_* \rho_\text{R}} \right]
      \]
  • 지금까지의 수식 전개에서는 genereal caloric EOS에 대한 어떠한 가정도 없었음.
    • Ideal caloric EOS를 가정하여, ideal gas에 적용하는 shock relations을 유도하면, density ratio $\rho_* / \rho_\text{R}$과 pressure ratio $p_* / p_\text{R}$ 사이의 유용한 관계식이 도출됨. \[
      \frac{\rho_*}{\rho_\text{R}} = 
      \frac{\left( \frac{p_*}{p_\text{R}} \right) + \left( \frac{\gamma - 1}{\gamma + 1} \right)}{\left( \frac{\gamma - 1}{\gamma + 1} \right) \left( \frac{p_*}{p_\text{R}} \right) + 1}
      \]
    • Mach number $M_\text{R} = u_\text{R}/a_\text{R}, M_\text{S} = S_3/a_\text{R}$를 도입하여, density ratio와 pressure ratio를 표현하면 다음과 같음. $$ \frac{\rho_*}{\rho_\text{R}} = \frac{(\gamma + 1)(M_\text{R} - M_\text{S})^2}{(\gamma - 1)(M_\text{R} - M_\text{S})^2 + 2} $$ $$ \frac{p_*}{p_\text{R}} = \frac{2\gamma(M_\text{R} - M_\text{S})^2 - (\gamma - 1)}{(\gamma + 1)} $$
    • Shock speed를 pressure ratio의 함수로 표현하면 다음과 같음. \[
      S_3 = u_\text{R} + a_\text{R} \sqrt{\left(\frac{\gamma + 1}{2\gamma}\right) \left(\frac{p_*}{p_\text{R}}\right) + \left(\frac{\gamma - 1}{2\gamma}\right)}
      \]
      • Shock strength가 0이 되면, pressure ratio는 1이 되기 때문에, shock speed $S_3$은 characteristic speed $\lambda_3 = u_R + a_R$이 됨.
    • Shock wave 이전의 particle velocity $u_*$를 아래의 식과 같이, density ratio로 표현할 수 있음. $$ u_* = (1 - \rho_\text{R}/\rho_*)S_3 + u_\text{R}\rho_\text{R} / \rho_* $$
    • $S_1$의 속도로 전파하는 1-shock wave에 대한 분석도 3-shock wave와 동일하게 처리할 수 있음. Stationary frame of reference로 변환하면, relative velocity와 mach number는 다음과 같음. \[
      \hat{u}_\text{L} = u_\text{L} - S_1, \quad \hat{u}_{*} = u_{*} - S_1
      \] $$ M_\text{L} = u_\text{L}/a_\text{L}, \quad M_\text{S} = S_1/a_\text{L} $$
      • Density와 pressure ratio 관계는 다음과 같음. $$ \frac{\rho_*}{\rho_\text{L}} = \frac{\frac{p_*}{p_\text{L}} + \frac{\gamma-1}{\gamma+1}}{\frac{\gamma-1}{\gamma+1}\frac{p_*}{p_\text{L}} + 1} $$
      • Left shock 전후의 density와 pressure ratios를 relative Mach number $M_\text{L} - M_\text{S}$로 표현하면 다음과 같음. \[
        \frac{\rho_*}{\rho_\text{L}} = \frac{(\gamma + 1)(M_\text{L} - M_\text{S})^2}{(\gamma - 1)(M_\text{L} - M_\text{S})^2 + 2}
        \] \[
        \frac{p_*}{p_\text{L}} = \frac{2\gamma(M_\text{L} - M_\text{S})^2 - (\gamma - 1)}{(\gamma + 1)}
        \]
      • Pressure ratio로부터 shock speed $S_1$를 다음과 같이 구할 수 있음. $$ M_\text{L} - M_\text{S} = \sqrt{\frac{\gamma + 1}{2\gamma}\frac{p^*}{p_\text{L}} + \frac{\gamma -1}{2\gamma}} $$ $$ S_1 = u_\text{L} - a_\text{L} \sqrt{\frac{\gamma + 1}{2\gamma}\frac{p^*}{p_\text{L}} + \frac{\gamma -1}{2\gamma}} $$
        • Shock strength가 0으로 가게 되면, $p_*/p_\text{L}$은 1이 되고, shock speed $S_1$는 characteristic speed $\lambda_1 = u_\text{L} - a_\text{L} $이 됨.
      • Left shock 이전의 particle velocity는 다음과 같음. $$ u_* = \left(1-\frac{\rho_\text{L}}{\rho_*}\right)S_1 + \frac{u_\text{L}\rho_\text{L}}{\rho_*} $$